0
\$\begingroup\$

I'm having troubles trying to rearrange a set of points where each point is part of the edge of a concave polygone. My set of points is not sorted in any order, they are just randomly put in an array. See the example right below : 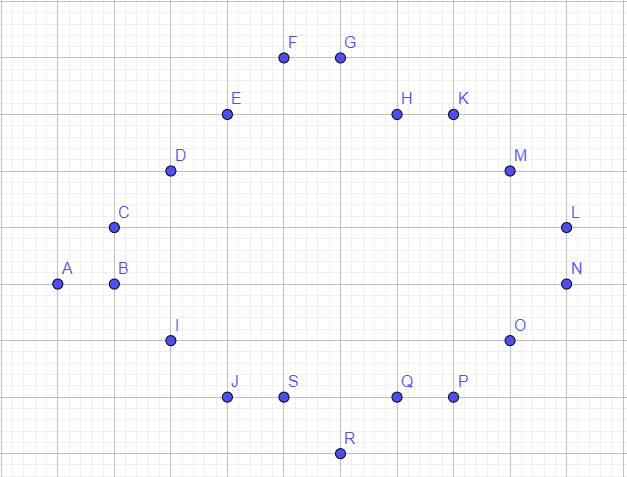enter image description here

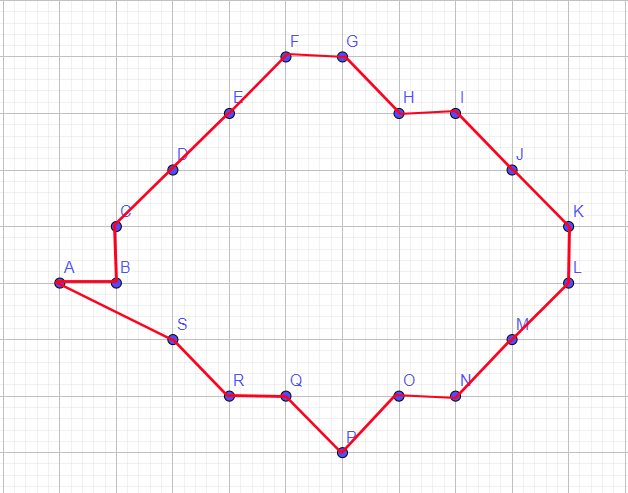
My goal is to rearrange these points to be able to build a polygon correctly where the next index in the array is the next point to draw : enter image description here

I don't know how to do it fast, sometime arrays are made of 200 points, and I have like 20 of these arrays (I would use this to draw islands)

Thank you in advance.

\$\endgroup\$
2
  • \$\begingroup\$ I don't think this is a well defined problem. E.g. in your example, going from I to B to A to C is an equally valid convex polygon as going I , A, B, C. \$\endgroup\$
    – Bram
    Commented Jan 10, 2019 at 1:25
  • \$\begingroup\$ Yes but it's not a problem as I want to find one of the concave polygone that may be build out of a set of points. \$\endgroup\$
    – Fosheus Badabu
    Commented Jan 10, 2019 at 9:13

1 Answer 1

Reset to default
1
\$\begingroup\$

Maybe sort them on angle of vector from average to point?

In pseudo code:

MID = AVERAGE(PTS)
ANGLES = []
FOR EACH PT:
    V = PT - MID
    ANG = ATAN2F( PT.Y, PT.X )
    ANGLES.APPEND( (ANG, PT ) )
SORT( ANGLES )
FOR ANG,PT IN ANGLES:
   OUTPUT(PT)
\$\endgroup\$
2
  • \$\begingroup\$ Thank you for your answer but I think this méthod does not work with concave polygon as I already tried it and it builds a convex polygon out of these points. In the example I provided it's not that easy to see. \$\endgroup\$
    – Fosheus Badabu
    Commented Jan 10, 2019 at 9:10
  • \$\begingroup\$ This looks workable to me if we can reliably place the center point in the polygon's interior, though I think we need some additional sorting by distance when we encounter a run of 3 or more points along a ray from our centroid (all at the same angle, to within some tolerance). If the average point lies outside the expected polygon (think of a very skinny crescent moon with narrow tips that almost wrap around) we'll get a less desirable result. This method implicitly assumes the polygon is "star-shaped" in the mathematical sense, which covers non-convex cases but not every concave polygon. \$\endgroup\$
    – DMGregory ♦
    Commented Jan 10, 2019 at 12:37

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .