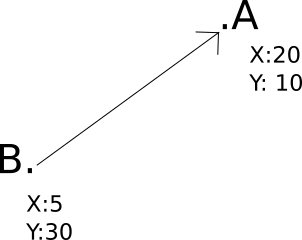
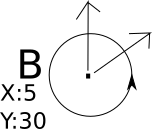First we get a vector from B to A, as in the following picture:
Now we have a vector that tells us how to get exactly from B to A. In code it looks something along these lines:
var BtoA = A - B;
In the above image's case, the resulting vector is X:15 Y:-20. At this point we actually already know the direction, as it is included in the information of how to get from B to A. Then if we need to know just the direction, we get that by normalizing the vector we have:
var direction = normalize(BtoA);
Normalizing a vector does this to it:
vec2 normalize(vec2 v)
{
return v / sqrt(v.x * v.x + v.y * v.y);
}
To break it down, first we get the length (aka magnitude) of the vector by Pythagorean theorem which you probably have learned at school. After we have the length of the vector, we divide the original vector by it's own length to make it a unit vector. In other words, we reduce the length of BtoA into just one, which makes it an unit vector.
Now we have a direction vector that looks like this:
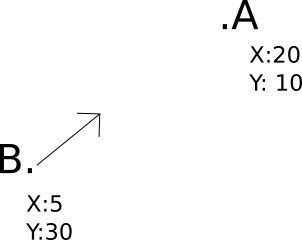
And the resulting unit vector is X:0.6 Y:-0.8. If you want to make sure, you can check it's length, which should be 1:
var v = vec2(0.6, -0.8);
var length = sqrt(v.x * v.x + v.y * v.y);
print(length); // Should print 1
Now at this point, the unit vector we got from normalizing the BtoA vector is now just pointing at the direction of A from the point of B.
Basically we have only reduced the length of the BtoA vector to 1, which is why it is just pointing at the direction of A from the viewpoint of B.
And then as you asked, how to figure out if person B is facing at the same direction of person A, you need to know the orientation of person B and A. You don't get that just by calculating the direction to A from B.

The orientation is found by first figuring out the orientation vector of B, which you can calculate if you have the rotation angle of B:
var rotation = 1.5708; // 90 degrees in radians, radians are easier in code
var orientationVec = vec2(sin(rotation), cos(rotation));
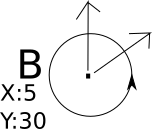
Now the orientation vector should be X:0 Y:1, because it is in 90 degree angle. Now that we have the orientation of B, we can check if it is facing A by checking the dot product:
var facing = dot(direction, orientationVec);
if(facing > 0)
print("Yes, the B vector is roughly facing A");
What is a dot product then? It looks like this:
float dot(vec2 a, vec2 b)
{
return a.x * b.x + a.y * b.y;
}
The dot product has a property of telling if two unit vectors are in parallel, and if not how much they differ. If the dot product is 1, the two unit vectors are pointing exactly at the same direction. If it is 0, the unit vectors are perpendicular to each other. So any values above 0 means that the unit vectors are going in roughly the same direction.
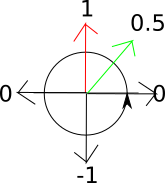
Of course using 0 as a threshold for determining if B is facing A is a bit wrong here, as you are talking about a person. If you define that a person is facing the other when the orientation towards the other is in 90 degree range, you can just check if the facing value is more than 0.5, which probably gives better results. You can also define that a person is facing another only when they are exactly looking at the other, which then requires you to use a value very close to 1.
So to put it all together:
bool IsBFacingA(vec2 A, vec2 B, float bRotation, float tolerance)
{
var BtoA = A - B;
var dir = normalize(BtoA);
var orientation = vec2(sin(bRotation), cos(bRotation));
var facing = dot(dir, orientation);
return facing >= tolerance;
}
Now you can tell if B is facing A. It requires a bit more than just the normalized direction from B to A.
But this still does not answer your question.
You can use all that information to determine if A and B are facing the same direction, by getting the orientation of both A and B and comparing them with the dot product. Like this:
bool IsBFacingTheSameDirectionAsA(float aRotation, float bRotation, float tolerance)
{
var aOrientation = vec2(sin(aRotation), cos(aRotation));
var bOrientation = vec2(sin(bRotation), cos(bRotation));
var facing = dot(aOrientation , bOrientation );
return facing >= tolerance;
}
And there you go, that is how you determine if A and B are facing the same direction.