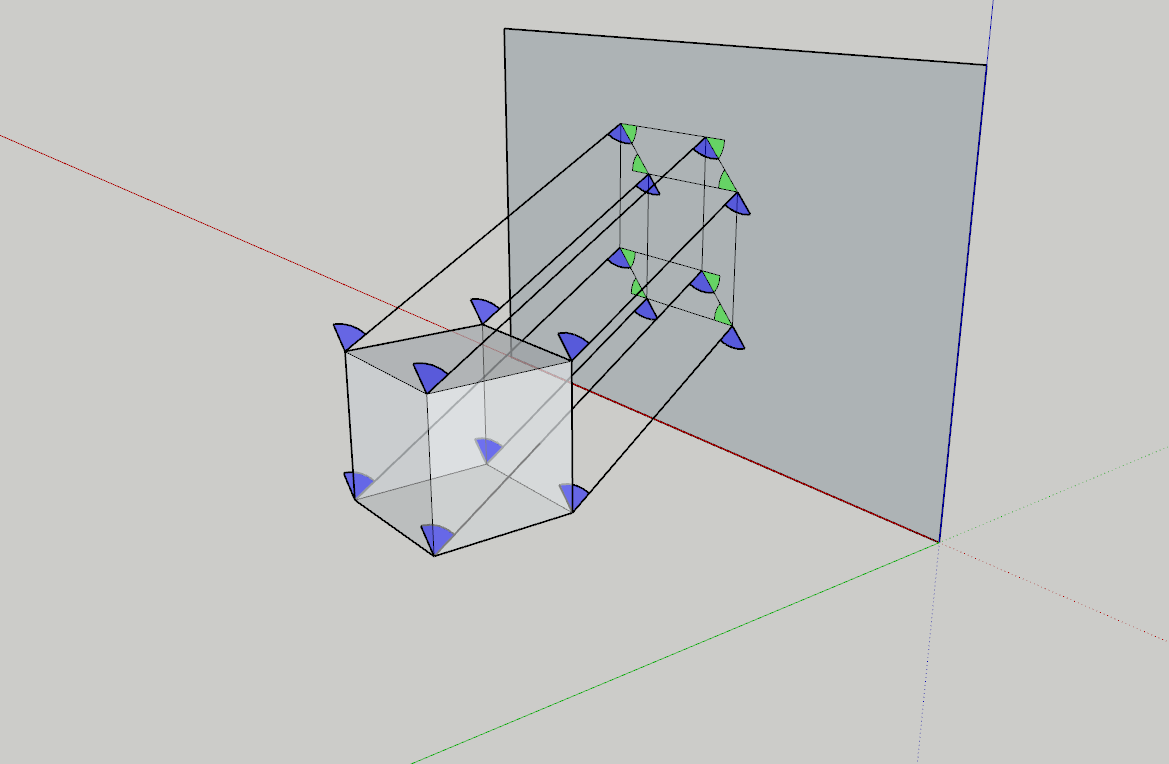
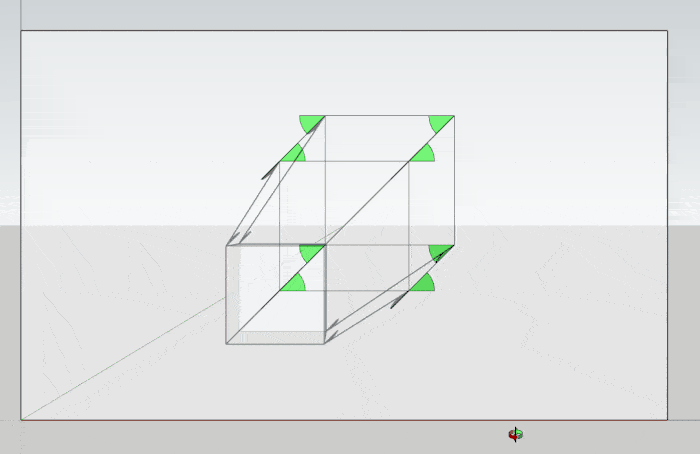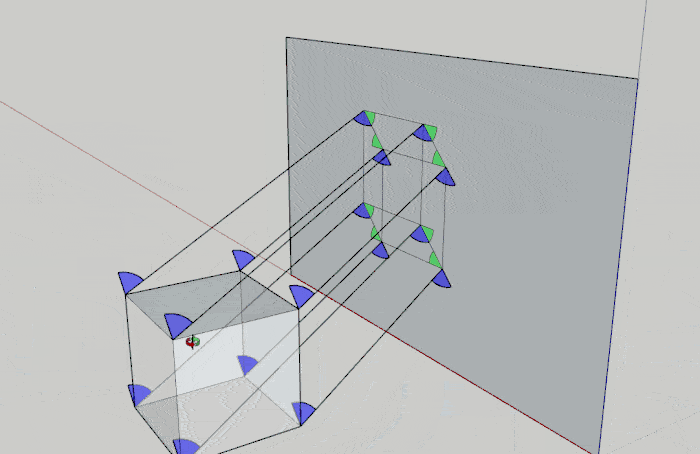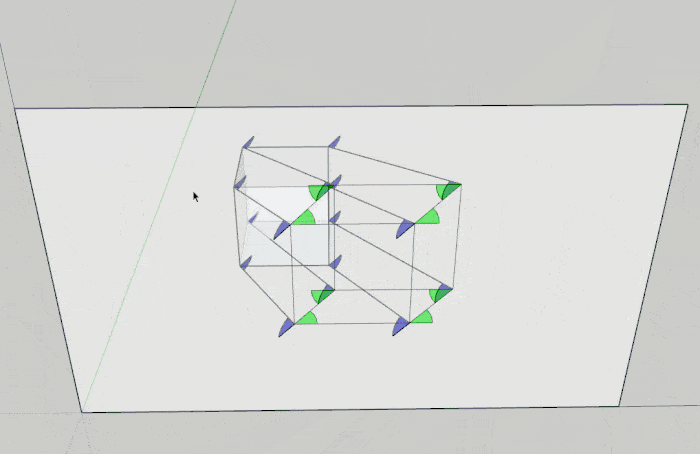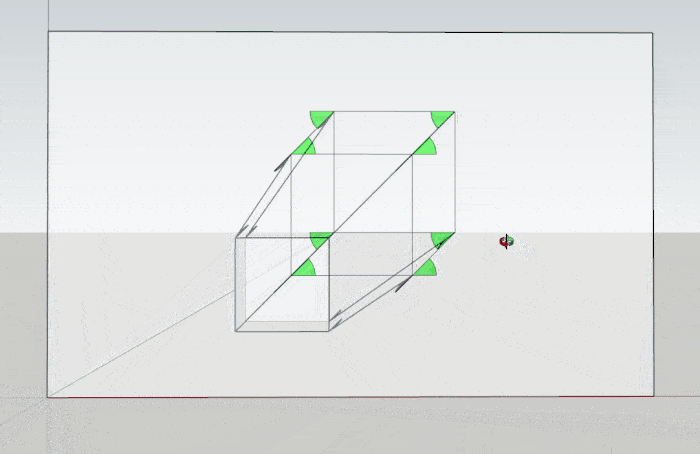
We know that in Oblique Parallel Projection Point (x,y,z) is projected to position (x_p,y_p) on the view plane.Projector (oblique) from (x,y,z) to (x_p,y_p) makes an angle alpha.
with the line (L) on the projection plane that joins (x_p,y_p) and (x,y).
Line L is at an angle phi with the horizontal direction in the
projection plane.See this image1:

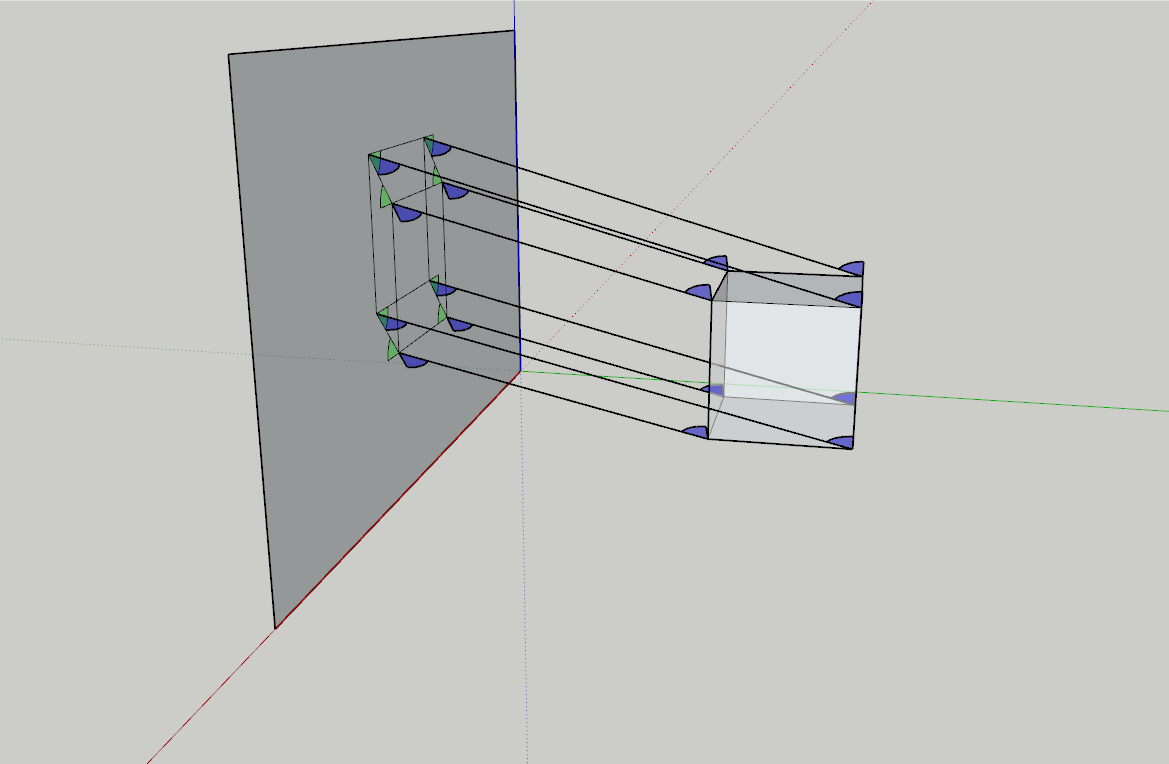
And in Oblique Parallel Projection Angles, distances, and parallel
lines in the plane are projected
accurately.For example see below image2: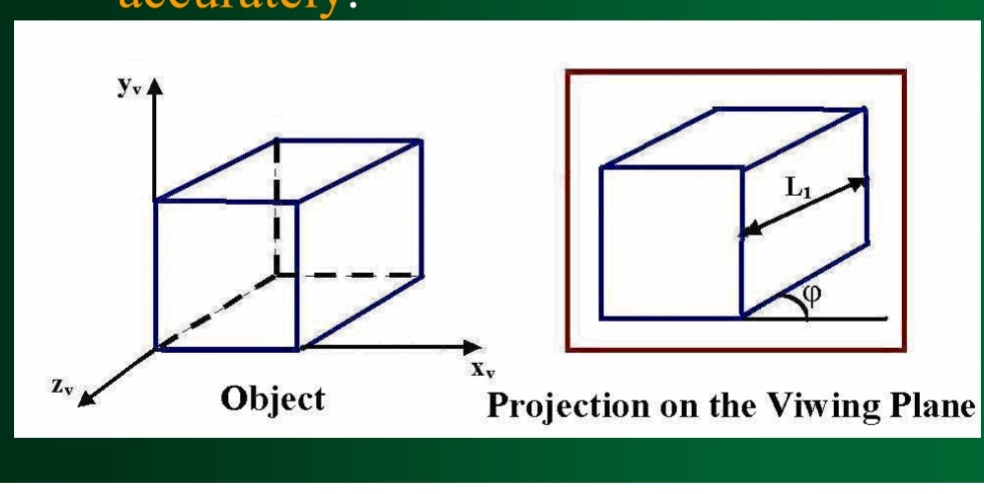
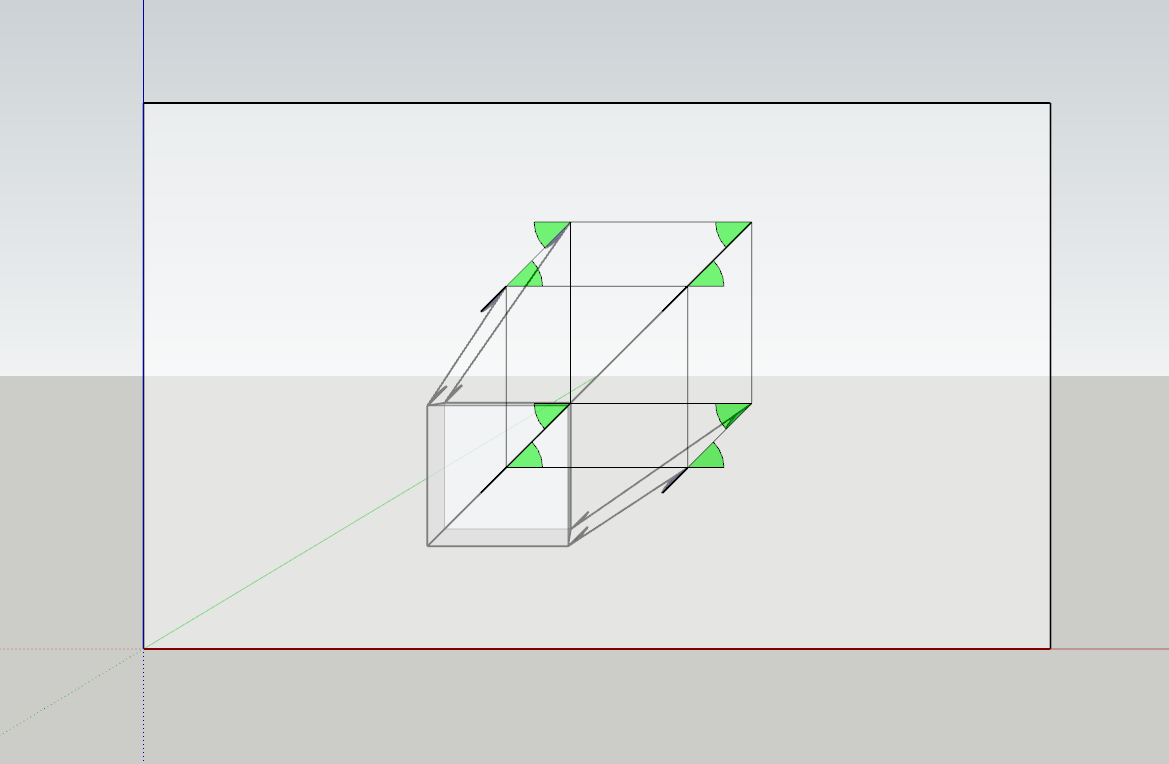
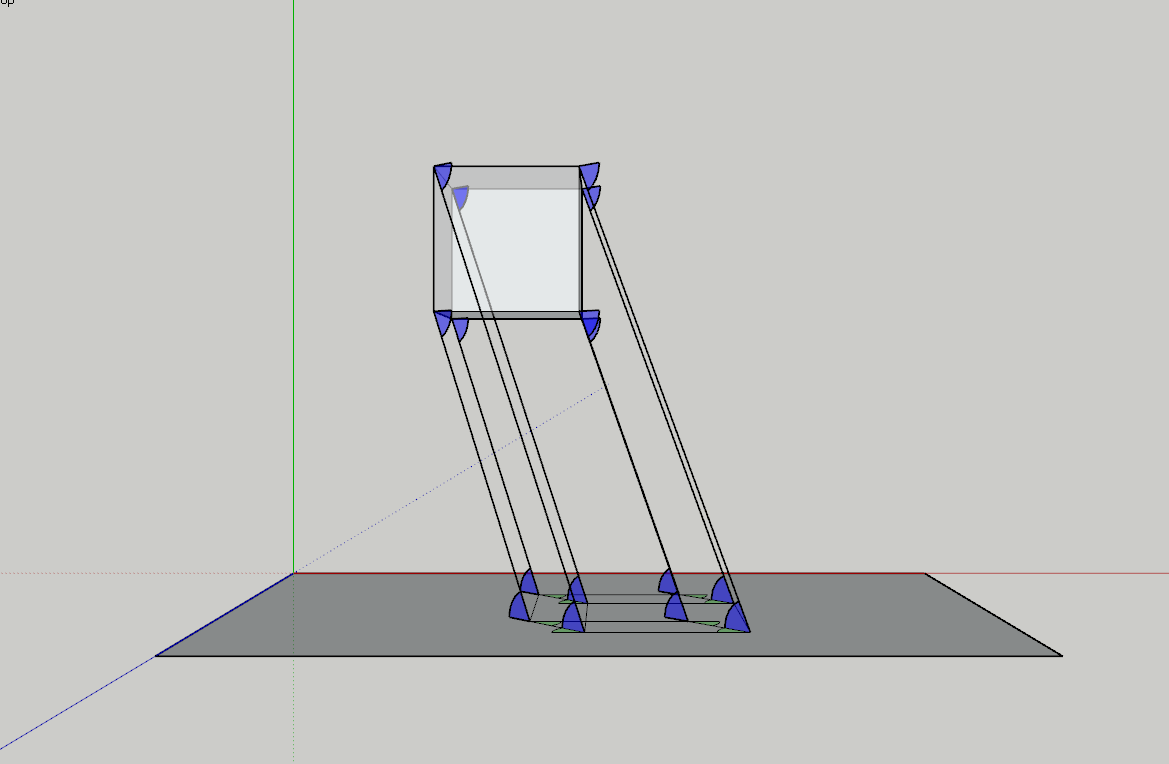
My question is where is the angle alpha in image2, I mean I see the angle phi on the image , so where is alpha in that image to understand better?I want to see 12 edges of original image projected to view plane with projector and angle alpha,phi levelling.
N. B:1 -- I am following Hearn and Baker book which screenshot like this.
N. B. -- I want to understand just intuition in easy way rather than details.