I'm currently in the process of making a 3d "semi" top down game, the camera comes in at a very high angle near 90 to show off the 3d models. I have a main character which can move in 4 axis (up, down, left right) using the arrow keys. I'm trying to create a smooth rotation between each of the axis so that when you press right when the character faces up, his rotation just doesn't jump.
Rotations seem to be weird and I don't quite understand why MathHelper.ConvertToDegrees or Radians doesn't quite give me what I want. When I try to rotate the model, I am translating it back to the origin, rotating then translating back for my order of matrices.
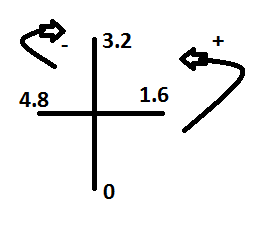
Although what I've found is that for rotation in the Z-axis, 0f seems to be down, 1.6f seems to be right, 3.2f seems to be up and 4.8f seems to be left. So to translate smoothly what I do is say I'm facing up (3.2f) and I want to face, right: Rotation.Z > 1.6 so, while it's greater keep subtracting 0.08f until we reach 1.6 or lower. This works well.
BUT my problem lies in since down is 0 and left is 4.8, when you're facing left and want to face down, you rotate clockwise all the way back to down instead of the logical counter-clockwise. This only happens in left to down or vice versa.
I've thought long and hard about this, down can also be expressed by 5.4f I assume but that doesn't really solve my problem. The approach for going down looks like this:
else if (currentKeyState.IsKeyDown(Keys.Down))
{
//Rotate character to face this direction
if (rotation.Z != 0)
{
if (rotation.Z < 0)
{
rotation.Z += 0.08f;
}
else if((rotation.Z > 0))
{
rotation.Z -= 0.08f;
}
}
Since for the left rotation, the rotation.Z value will be 4.8, then by this logic it'll going counter-clockwise. It's probably a simple solution but it has me stumped and one of my biggest problems is not moving on until a problem is fixed even if the game still works.
I've attached a picture of the axis just in case my post is nonsensical:
Look forward to any potential solutions.
