10
\$\begingroup\$

I am working on a tiled map editor, and I need to choose tiles automatically, based on the adjacent tiles. For example, when placing a road tile, next to another road tile, the two need to be oriented so that they form a continuous road. If there are other roads around them, we may need to use corner or intersection tiles.

Can anyone recommend some algorithms for doing this? The game uses an 8-directional square tiled map.

\$\endgroup\$
2
  • \$\begingroup\$ Can't you just examine all the surrounding 8 tiles whenever the user puts down a new tile, and the orient the new tile accordingly? Of course you'll need to store some extra info on each tile such as orientation and type. \$\endgroup\$
    – XiaoChuan Yu
    Commented May 23, 2012 at 22:18
  • \$\begingroup\$ I am examining all surrounding tiles, but I didn't know how to handle all the tile combinations. For example, you have seven possible tiles to choose from (horizontal, vertical, four corners, and a cross). I thought of using complicated switch statements, but that felt wrong. \$\endgroup\$
    – alekop
    Commented May 23, 2012 at 23:02

2 Answers 2

Reset to default
18
\$\begingroup\$

Maybe this is how it's typically done. You have your list of different tiles that represent a road tiles in all their possible orientations. Left to right, all four corners, top to bottom, whatever. Now you'll index all those tiles with a byte each. 8 bits, one for each direction. This could be in a hashmap or by file name... however you want to do this.

So you have this:

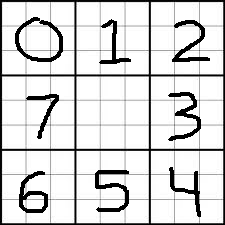
enter image description here

The byte code for the above tile is 00000000. Then your tile that goes from left to right (or right to left) is like this:

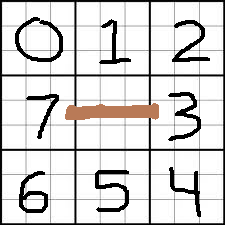
enter image description here

The byte code for that tile is 10001000, or 136. As another example, lets look at a three way intersection:

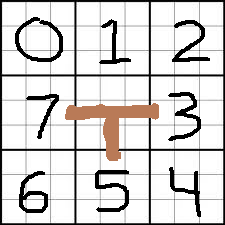
enter image description here

The byte code for that tile is 10101000.

You probably see where I'm going. You set bit positions in the byte representing connections. This is far better than trying to do some big if/else chain that I've seen before. When you're looking to place a tile, examine the tiles around it and create a byte along the way. Set 1's for tiles that have roads (or whatever you're looking to connect) and 0's for tiles that don't. When you're done, you'll have the byte code for the exact tile you need.

Note that when creating the assets you can reuse a lot of them by simply rotating and assigning the correct byte code to it.

EDIT: Updated images to be less crappy. Yes those are better than before.

\$\endgroup\$
8
  • \$\begingroup\$ Very nice! Simple and efficient. The only thing that I don't understand is how you're getting those bitmasks. For example how are you getting a bitmask of 17 from the numbers 3 and 7? \$\endgroup\$
    – alekop
    Commented May 23, 2012 at 22:41
  • \$\begingroup\$ Never mind, I see what you're doing. You're setting bits 3 and 7, but you're counting from the left, instead of the right. \$\endgroup\$
    – alekop
    Commented May 23, 2012 at 22:46
  • \$\begingroup\$ Oh wow, how embarrassing. Got my Endianness all mixed up. That was an accident I'll fix that! \$\endgroup\$
    – House
    Commented May 23, 2012 at 22:48
  • \$\begingroup\$ Great, now my comment doesn't make sense! :p Just kidding, thanks for your answer. That's exactly what I was looking for. \$\endgroup\$
    – alekop
    Commented May 23, 2012 at 22:54
  • 1
    \$\begingroup\$ Good explanation. I have a blog post covering the same technique with actual code and tile resolution: kitsu.github.io/2016/07/18/roguelike-project-05 \$\endgroup\$
    – kitsu.eb
    Commented Jul 19, 2016 at 17:48
3
\$\begingroup\$

I would recommend you take a look at this handy page for more information, as it goes into great detail about pretty much every aspect of what you're doing, as well as a few potential optimizations: http://www.angryfishstudios.com/2011/04/adventures-in-bitmasking/

The tldr is that you query each adjacent cell and store the combination in a bitfield/byte, then pass it through a map that converts a number from 0~255 into a value from 0~47, which corresponds to a unique image.

\$\endgroup\$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .